How many sig figs in 90 – How many significant figures in 90? This seemingly simple question unveils a captivating journey into the realm of numerical precision, where every digit holds a story and every zero has a purpose. Delve into this discourse as we unravel the enigmatic rules governing significant figures, illuminating the path to accurate calculations and scientific understanding.
In the realm of science and engineering, the concept of significant figures plays a pivotal role in ensuring the accuracy and reliability of our measurements. By mastering the art of identifying and counting significant figures, we gain the ability to express numerical values with clarity and confidence.
Introduction
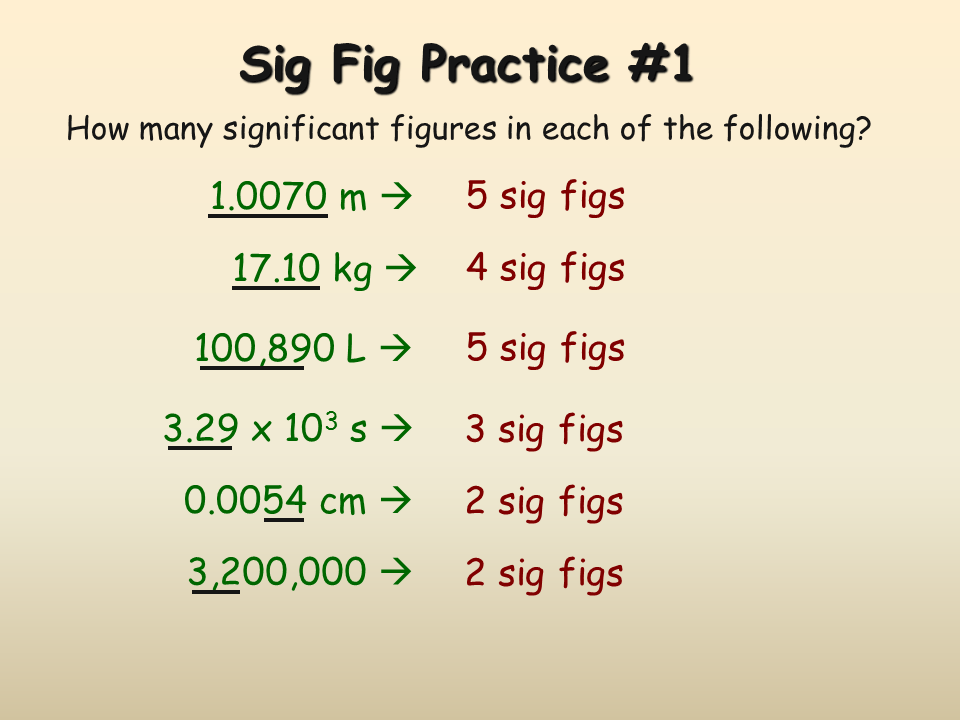
Significant figures (sig figs) are the digits in a number that are known with certainty, plus one uncertain digit. They provide a way to express the precision of a measurement.
There are three rules for determining sig figs:
- All non-zero digits are significant.
- Zeros between non-zero digits are significant.
- Trailing zeros after a decimal point are significant.
Zeros
Zeros can be tricky, so here are some additional rules:
- Leading zeros (zeros before the first non-zero digit) are not significant.
- Zeros used as placeholders (to show the location of the decimal point) are not significant.
Applying the Rules to “90”

Let’s analyze the number “90” using the rules for significant figures.
Identifying Non-Zero Digits
“90” has two non-zero digits
9 and 0.
Determining Significant Zeros
The zero in “90” is not significant because it is a trailing zero. Trailing zeros are not significant unless they are between non-zero digits.
Explanation
Since the zero in “90” is not significant, “90” has only one significant figure, which is the digit 9.
Examples and Non-Examples
To illustrate the concept of significant figures, let’s examine some examples and non-examples of numbers with different numbers of significant figures.
Examples
- 12.00has foursignificant figures. The zeros after the decimal point are significant because they indicate the precision of the measurement.
- 0.0050has twosignificant figures. The zeros before the first non-zero digit are not significant because they do not contribute to the precision of the measurement.
- 1.23 x 10-3has threesignificant figures. The exponent of the scientific notation does not affect the number of significant figures.
Non-Examples, How many sig figs in 90
- 100has onesignificant figure. The zeros after the non-zero digit are not significant because they do not indicate the precision of the measurement.
- 0.05000has fivesignificant figures. The trailing zeros after the last non-zero digit are not significant because they do not contribute to the precision of the measurement.
| Number | Significant Figures | Reason |
|---|---|---|
| 12.00 | 4 | Zeros after decimal point indicate precision |
| 0.0050 | 2 | Zeros before first non-zero digit are not significant |
| 1.23 x 10-3 | 3 | Exponent does not affect significant figures |
| 100 | 1 | Zeros after non-zero digit are not significant |
| 0.05000 | 5 | Trailing zeros after last non-zero digit are not significant |
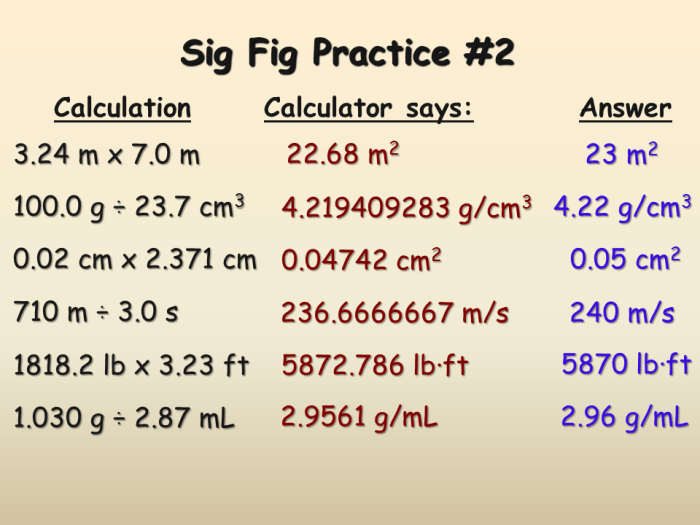
Applications in Calculations

Significant figures play a crucial role in calculations, ensuring the accuracy and precision of our results.
The number 90 has two significant figures, since the zeros are placeholders. For more information on significant figures, check out the barf bag lab answer key . Returning to our original topic, this means that 90 has two significant figures, which are 9 and 0.
When performing calculations involving numbers with different numbers of significant figures, we round the final answer to the least number of significant figures present in the original numbers.
Rounding Numbers Correctly
- If the first digit to be dropped is less than 5, the last retained digit remains unchanged.
- If the first digit to be dropped is greater than 5, the last retained digit is increased by 1.
- If the first digit to be dropped is 5 and the last retained digit is even, the last retained digit remains unchanged.
- If the first digit to be dropped is 5 and the last retained digit is odd, the last retained digit is increased by 1.
Examples of Calculations Involving Significant Figures
- 2.34 + 5.6 = 8.0 (2 significant figures)
- 12.34 x 0.05 = 0.62 (3 significant figures)
- (2.34 + 5.6) / 3 = 2.7 (2 significant figures)
Advanced Considerations

Determining significant figures can become more nuanced in certain situations. Here are some advanced considerations to keep in mind:
Exceptions to the Rules
There are a few exceptions to the general rules for determining significant figures. These exceptions include:
- Zeros to the left of a non-zero digit are not significant.
- Zeros between non-zero digits are significant.
- Zeros to the right of a decimal point, but not to the right of a non-zero digit, are significant.
For example, the number 0.005 has two significant figures (5 and 0), while the number 500 has three significant figures (5, 0, and 0).
Implied Decimal Points
When a number is written without a decimal point, it is assumed to have an implied decimal point at the end of the number. For example, the number 5 is equivalent to 5.0, which has one significant figure.
Scientific Notation
When a number is written in scientific notation, the number of significant figures is determined by the number of digits in the coefficient, not including the zeros used to place the decimal point.
FAQ Insights: How Many Sig Figs In 90
Why are significant figures important?
Significant figures are essential for ensuring the accuracy and reliability of scientific measurements and calculations. By understanding the concept of significant figures, we can express numerical values with precision and clarity, enabling us to make informed decisions and draw meaningful conclusions from our data.
How do I determine the number of significant figures in a number?
To determine the number of significant figures in a number, follow these rules:
What are some examples of numbers with different numbers of significant figures?
Here are a few examples of numbers with different numbers of significant figures:
